
Investigating Terao's freeness conjecture with computer algebra
Veröffentlicht am 21.04.2021
Lukas Kühne
In computational mathematics, one often encounters the problem of scanning (finite but) large sets of certain objects. Here are two typical scenarios:
- Searching for a counter-example of an open conjecture among these objects.
- Building a database of such objects with some of their invariants.
Recognized patterns and questions which a database answers affirmatively may lead to working hypotheses or even proofs by inspection. This research brief describes an instance of this principle in an ongoing research project with Mohamed Barakat in which we investigate hyperplane arrangements through a database [1, 2, 3].
A hyperplane arrangement is a finite set of hyperplanes containing the origin in some ambient vector space. The theory of hyperplane arrangements naturally lies at the crossroads of algebra, combinatorics, algebraic geometry, representation theory, and topology. We are specifically interested in the interplay between the combinatorial and algebraic properties of an arrangement motivated by Terao's freeness conjecture.
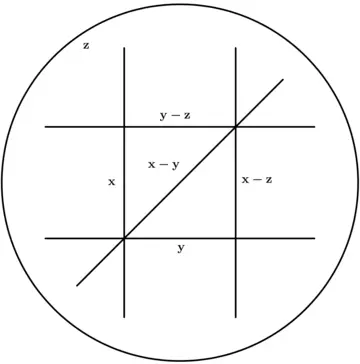
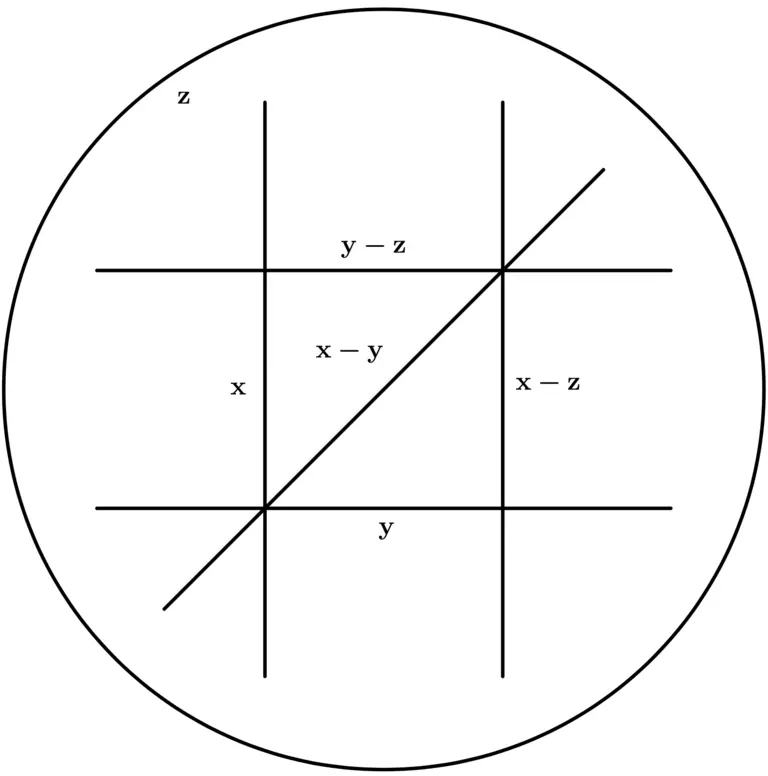
As an example, let us consider the braid arrangement \(A_3\) is an arrangement in \(\mathbb{R}^3\) defined via the linear equations \(x=0, y=0, z=0, x-y=0,x-z=0,y-z=0.\) Figure 1 shows a projectivized picture of \(A_3\), that is a slice of the arrangement with the hyperplane \(z = 1\). The hyperplane \(z = 0\) is shown as a circular line at infinity.

Combinatorially, an arrangement gives rise to a matroid. In general, a matroid is an abstraction of linear independence in vector spaces. In our case, it can be thought of as a graph that records incidences between the hyperplanes and the lower-dimensional subspaces, which arise as intersections of these hyperplanes.
Motivated from singularity theory, K. Saito introduced the notion of a logarithmic derivation of a hypersurface. Terao subsequently pioneered the study of such logarithmic derivation in the special case of hyperplane arrangements. These derivations are vector fields which are parallel to all involved hyperplanes. The collection of all derivations forms an algebraic structure called a module. In the case of the braid arrangement considered above, this module of derivations is free; that is, it admits a basis like in the case of vector spaces. In this case, we call the arrangement free. It turns out that in a nice situation, such as when the hyperplanes are the symmetry hyperplanes of a crystal-like structure, the arrangement is always free.
Over the years, remarkable connections were established between the freeness of an arrangement and related arrangement properties. However, it is fair to say that it is still quite mysterious to understand which arrangements are free and which are not. This is manifested in the main open conjecture in this area formulated by Terao about \(40\) years ago which asks whether the freeness of the derivation module over a fixed field is determined by the underlying matroid.
Our strategy to investigate this conjecture in dimension \(3\) consists of the following three steps:
- We generated all possibly relevant matroids in dimension \(3\) with up to \(14\) elements using a parallelized algorithm in \(\textsf{HPC-GAP}\), the high-performance computing version of \(\textsf{GAP}\). These are \(815 107\) matroids. We saved them in a public database.
- For each such matroid, we determined all corresponding hyperplane arrangements.
- We checked whether the arrangements coming from one matroid are either all free or nonfree.
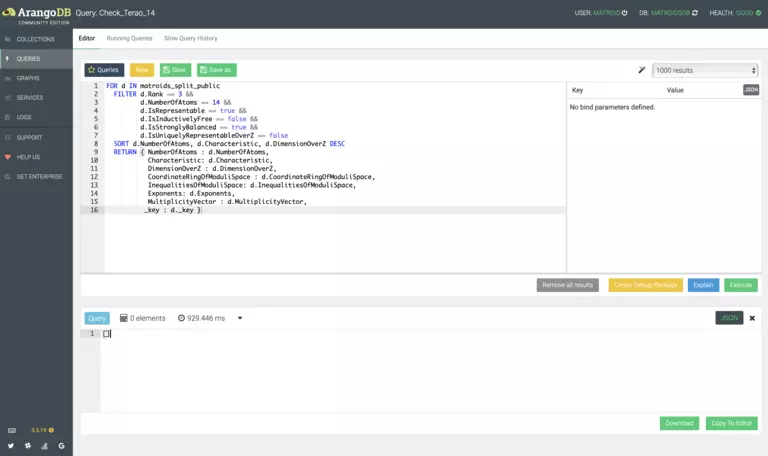
This approach enabled us to verify Terao's freeness conjecture for the first time for rank \(3\) arrangements with up to \(14\) hyperplanes. Figure 2 shows a screenshot of the query in our database of the matroids of size \(14\) that filters out all matroids that are not relevant for Terao's conjecture. As shown at the bottom of the figure, this query does not yield any matroid.
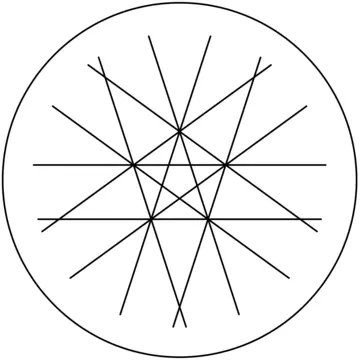
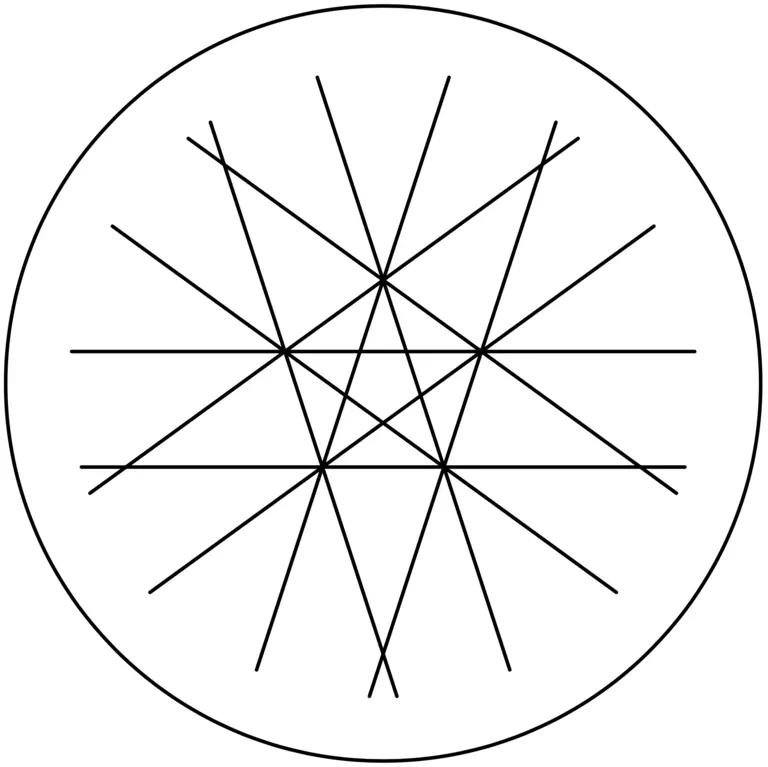
Interestingly, we found a new example of a matroid whose freeness is dependent on the underlying field. This is the so-called pentagon arrangement depicted in Figure 3. All corresponding hyperplane arrangements are free in all fields of characteristic not equal to \(2\) but nonfree over fields of characteristic \(2\).
References
| [1] | Mohamed Barakat, Reimer Behrends, Christopher Jefferson, Lukas Kühne, and Martin Leuner. On the generation of rank 3 simple matroids with an application to Terao's freeness conjecture, to appear in SIAM Journal for Discrete Mathematics. MIS-Preprint: [88/2020] |
| [2] | Mohamed Barakat and Lukas Kühne. \(\mathtt{matroids\_split\_public}\) – a database collection for rank \(3\) integrally split simple matroids, 2019. MatroidGeneration on GitHub |
| [3] | Mohamed Barakat and Lukas Kühne. Computing the nonfree locus of the moduli space of arrangements and Terao's freeness conjecture, in preparation. |
