Micromagnetics
Simple energy...
The Landau-Lifshitz model for ferromagnetic samples Ω states that the magnetization m, a vector field of unit length on Ω, is determined through the competition of four different mechanisms:
- The Exchange interaction causes the magnetization to prefer a common orientation throughout the whole sample.
- Via Maxwell's equations, the divergence of m and its normal component on the sample surface induce a curl free magnetic stray-field in the sample and the space surrounding it. The magnetization orients to minimize this field.
- The material may be anisotropic, e.g. in the sense that its crystalline structure creates certain "easy axes", to which the magnetization prefers to align.
- The ferromagnetic sample may be exposed to an external magnetic field. The magnetization then tries to align with the field.
The configurations that are observed in experiments are conjectured to be (local) minimizers of the associated Landau-Lifshitz energy E(m). We refer to [1] for details on this energy functional.
...but complex patterns
Through the four competing effects and the non-convex unit-length constraint, minimizers of E exhibit a large variety of interesting patterns, e.g.
When material parameters and involved length scales are extremal, one can often use (rigorous) asymptotic analysis to derive from the few basic effects mentioned above a reduced model that - in this particular regime of parameters - still captures the important features of the full Landau-Lifshitz model. Such a simplified model then will at best already explain the physical observations, or at least reduce the complexity of their numerical simulation.
Below, we give four examples that demonstrate the use of theoretical analysis, numerical simulation and experiments in order to explain certain physical phenomena:
Ferromagnetic materials can be divided into two classes with distinct features, depending on the strength of their anisotropic behavior. In our analysis, we usually assume that there is only one easy axis that is given by a unit-length vector \(e\), that is, the material is uniaxial.
Domain branching
In "hard" materials, where the contribution from the anisotropy dominates the Landau-Lifshitz energy \(E\), the magnetization essentially favors only two states: Parallel or anti-parallel alignment with the easy axis \(e\). Assuming a bulk sample that is not affected by an external magnetic field, the lower-order contributions from stray-field and exchange energy determine the actual pattern. While a constant magnetization \(m = e\) or \(m = -e\) avoids the exchange energy, the resulting stray-field is rather large, due to the non-vanishing normal component of \(m\) on a part a the sample surface. On the other hand, the stray-field energy, which can be expressed as \(H^{-1}\)-norm of \(div(m)\), favors magnetizations quickly oscillating between the two states \(+e\) and \(-e\). This, however, induces a large exchange energy.
Hence, the optimal configuration should be constant in the sample interior, where no stray field is generated, and refine towards the sample surface, where a quickly oscillating magnetization minimizes - as an electrostatic analogy - "surface charges", and thus the stray field. The speed of oscillations and refinement towards the sample surface is limited by the strength of the exchange energy.
We rigorously investigated this "domain branching" in a work with Viehmann [2], where the asympotically exact scaling of the minimal energy was derived, improving on previous work with Choksi and Kohn.
Wall configurations
However, one should note that the exchange energy in fact prevents a jumping magnetization, as was assumed in the previous heuristic reasoning. Indeed, it will rotate smoothly between two domains of almost constant magnetization, but on a very small length scale, compared to the size of the domains.
The type of those transition layers - usually called domain walls - is particularly interesting in the class of soft materials with almost negligible anisotropy, when additionally the sample is a thin ferromagnetic film: If the film is very thin, the exchange energy prevents variation of m in the thickness direction, while the stray-field energy prevents an out-of-film-plane component of \(m\) on the film surface, and hence also in the interior of the film.
By concentrating the exchange energy in a narrow core of fast rotation, while spreading the "magnetic charges" over long, logarithmically decaying tails, the optimal transition layer - called "symmetric Néel wall - further reduces its energy.
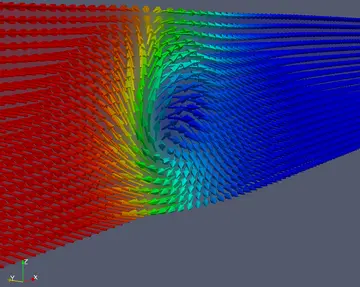
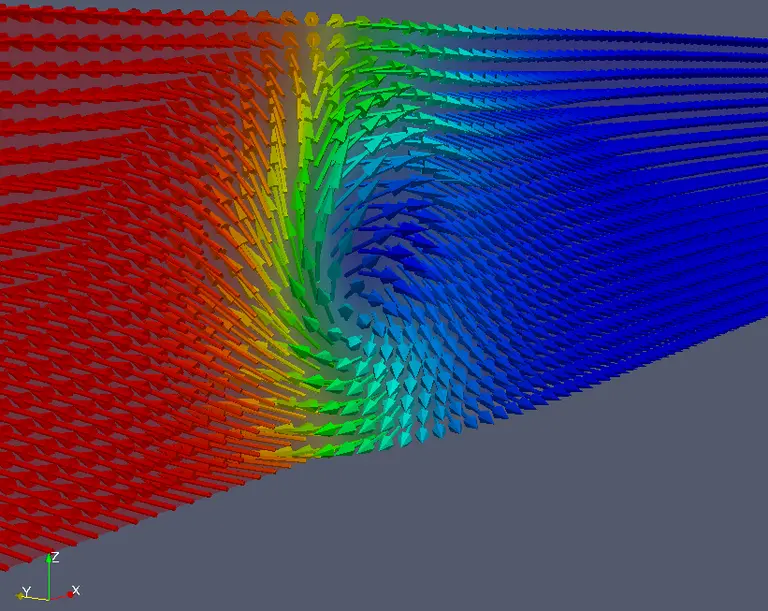
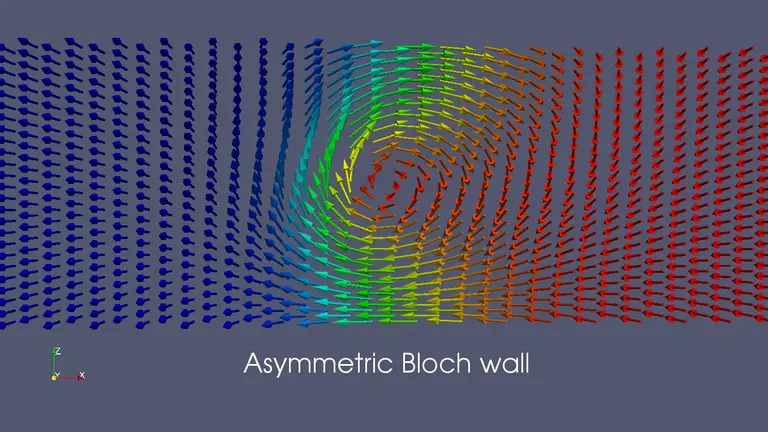
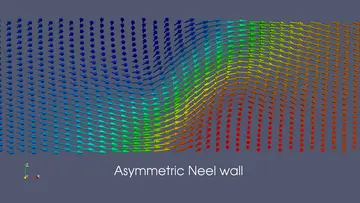
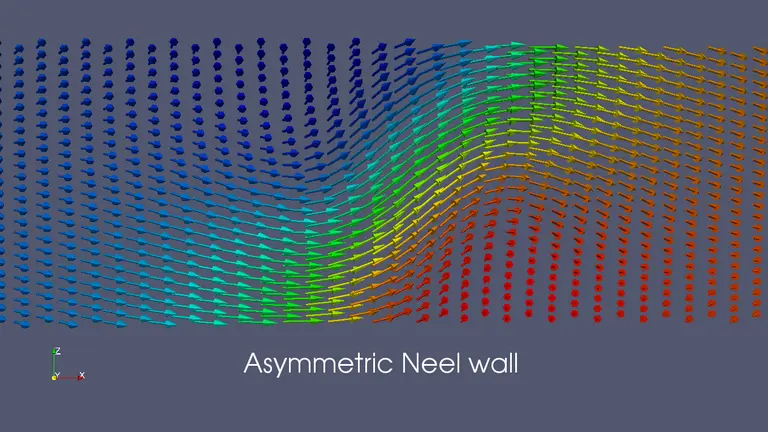
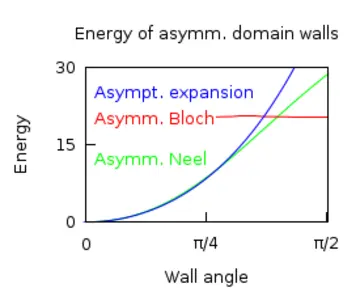
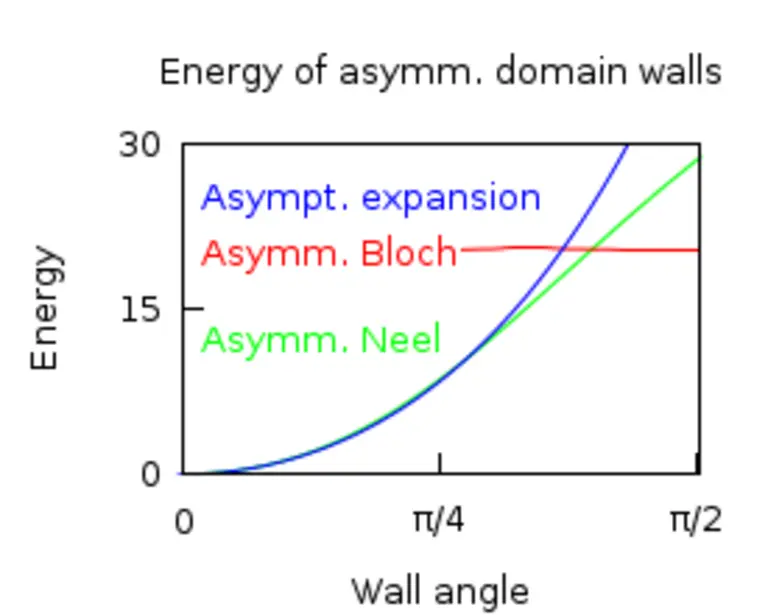
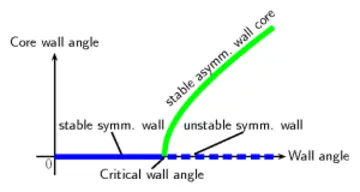
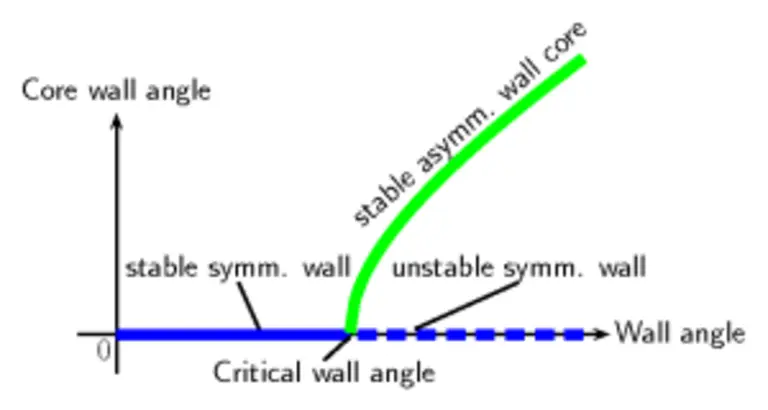
In thicker films, variation in the thickness direction is not as strongly penalized, such that two different divergence-free transition layers - the so-called asymmetric Néel and Bloch wall - can emerge. Those asymmetric walls are of small wall width and completely avoid the stray-field.
In the interesting regime of moderate film thickness, where symmetric and asymmetric domain walls are of comparable energy, the optimal transition layer is expected to exhibit both features: Logarithmically decaying tails are attached to asymmetric, divergence-free wall cores. With Ignat in [3], we derived a reduced model that captures the splitting of the wall energy into the energy of an asymmetric wall and the well-understood energy of the logarithmic tails. By exploiting the scale separation in narrow core and long tails, the reduced model is capable of determining the experimentally hardly accessible relative amount of rotation in the asymmetric wall core via numerical simulation of much lower complexity, compared to the full Landau-Lifshitz model.
By rigorously deriving a Taylor expansion of the mathematically challenging exchange (better known as Dirichlet) energy of divergence-free unit-length vector fields for small rotations in [4], we were able to give evidence of a supercritical bifurcation of an asymmetric wall core from the purely symmetric Néel wall structure, as film thickness and amount of rotation in the transition layer increase.
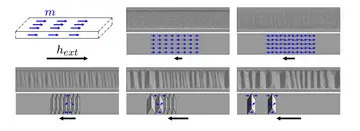
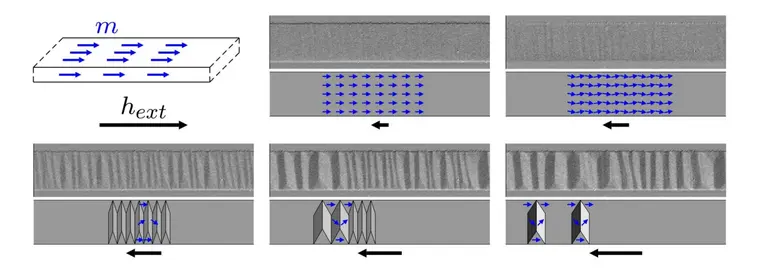
The cost of transition layers between domains of constant magnetization in terms of their energy per interfacial area strongly affects the observed domain patterns. An interesting configuration in very thin films, where the symmetric Néel wall is the favoured transition layer, is the so-called concertina pattern.
The concertina pattern
In a series of articles [5,6,7,8] and in collaboration with a group of experimental physicists of the IfW in Dresden, we investigated the formation and hysteresis of the concertina pattern.
This almost periodic magnetization pattern is frequently observed during the switching process of the magnetization in elongated thin ferromagnetic samples. The configurations observed during the switching, which is initiated by the slow reversal of an applied magnetic field, are meta-stable. This means that they are not ground-states but that they are local minima of the micromagnetic energy - as is typical for hysteretic processes.
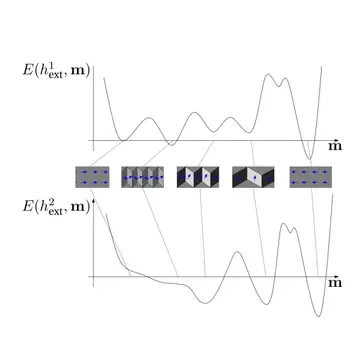
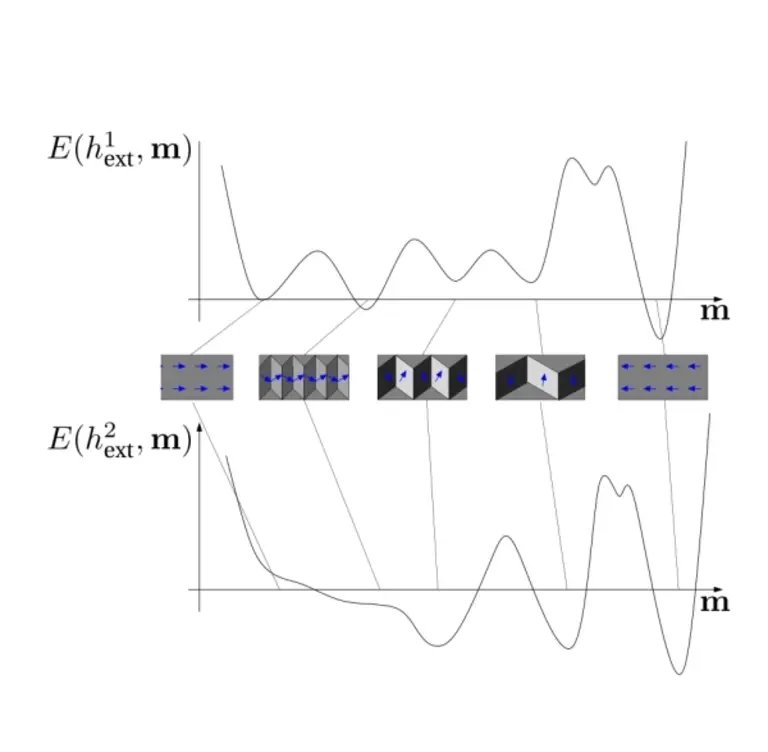
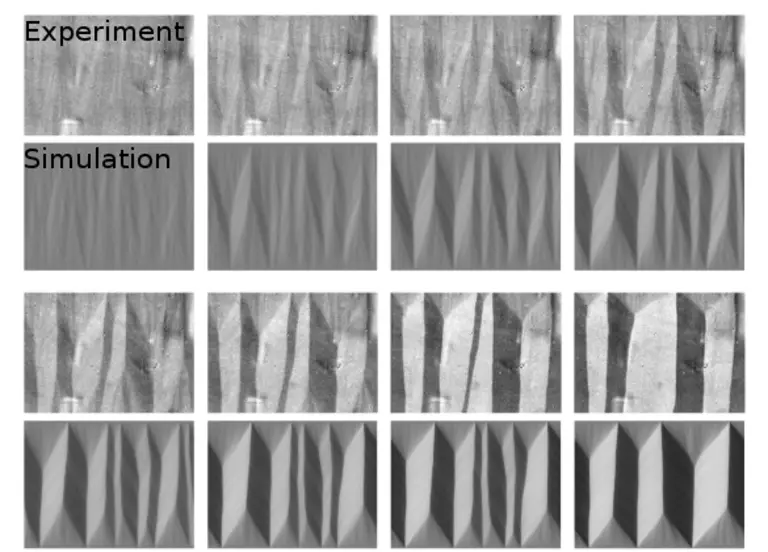
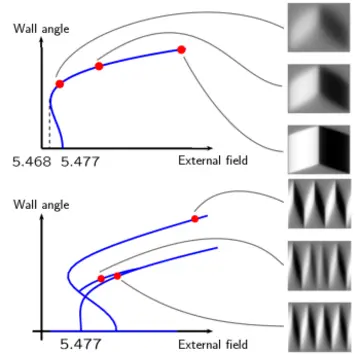
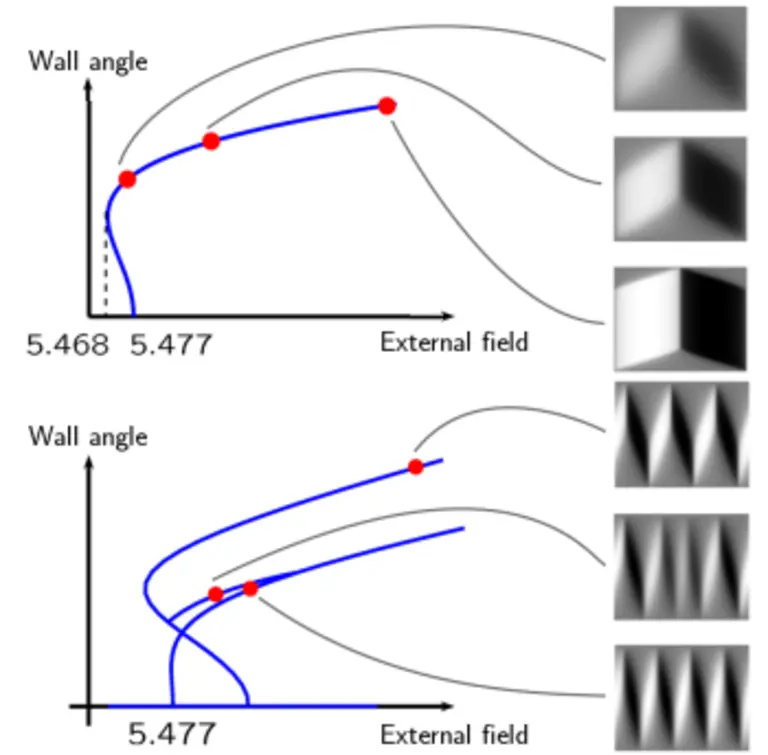
In the experiments, one observes that during its lifetime and before its decay the concertina pattern coarsens several times, successively increasing the period. Figure 2 schematically depicts the energy landscape for two different values of the external field. As the external field increases and passes the critical field, the energy landscape is deformed. Local minima are destroyed and the concertina pattern successively coarsens towards a pattern of large period.
The first step towards understanding this behavior was a linear stability analysis of the uniform magnetization by which we identified the relevant thin-film parameter regime. It turned out that at some critical value of the reversed applied field, the uniform magnetization becomes unstable under an oscillatory perturbation. The experimentally observed period of the oscillation is in relatively good agreement with the period of the unstable mode but always slightly larger. The basis for the identification of the relevant physical mechanisms behind this deviation and the coarsening of the pattern is a reduced model that we rigorously derived in [7]: Asymptotic analysis and numerical simulations revealed that both effects are related to a modulation instability which in turn is closely related to the so-called Eckhaus-instability - an instability that is known from the analysis of convective systems.
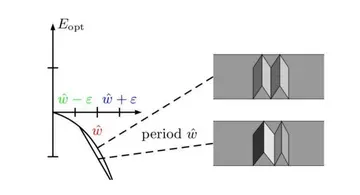
The mechanism of this instability can be understood on the level of domain theory which shows that the minimal energy per period is a concave function of the period. Hence, as depicted in Figure 3, it is energetically favorable to perturb a configuration consisting of two folds of equal width by slightly decreasing and slightly increasing, respectively, the width of the folds. This finally leads to the coarsening, see Figure 1.
Asymptotic analysis in the form of non-linear interpolation estimates and an extended bifurcation analysis allowed us to deduce the optimal and marginally stable period of the pattern as a function of the external field. In particular, the extended bifurcation analysis provides us with an understanding for the deviation of the period close to the critical field.
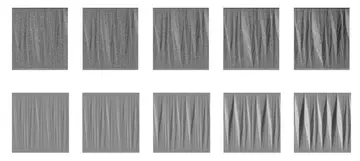
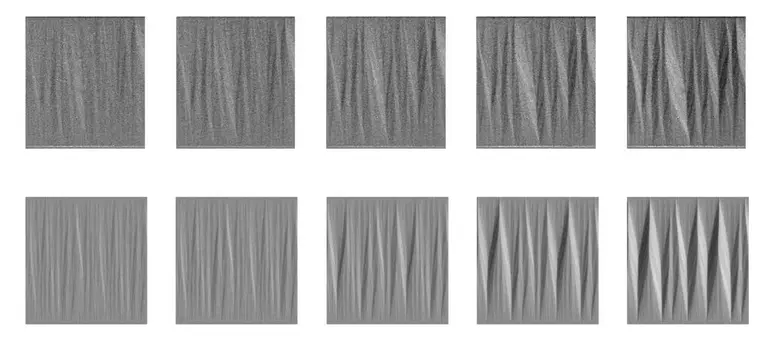
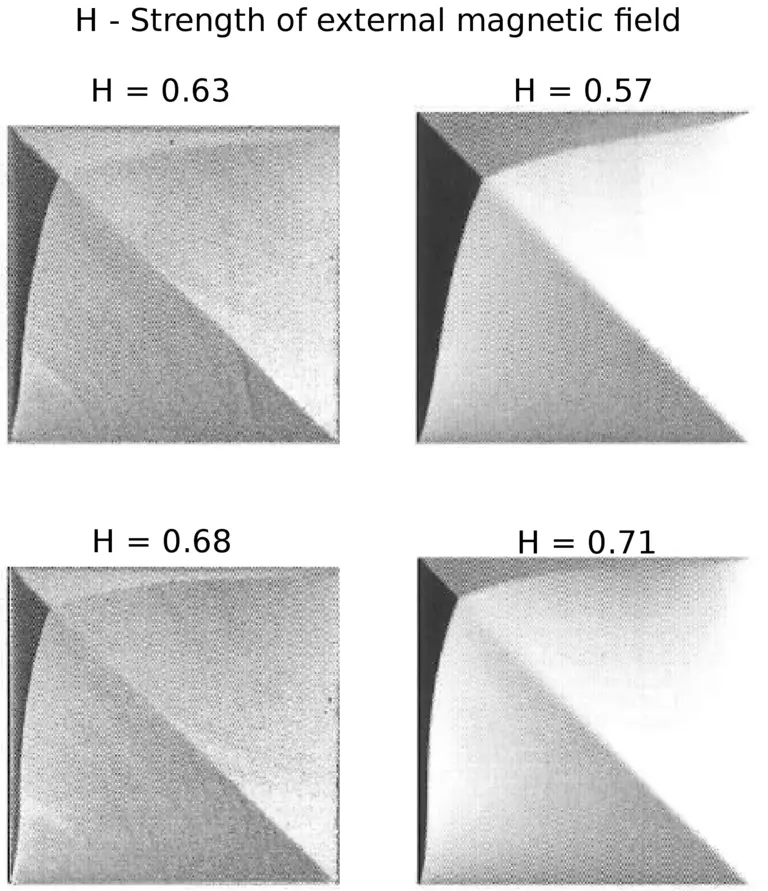
We also investigated the effect of material disorder, more precisely polycrystalline anisotropy: Figure 4 shows a comparison between the experimentally observed concertina and our simulations for different values of the external field.
Domain configurations in soft films of moderate aspect ratio
In [9] a reduced model for the simulation of soft ferromagnetic samples under an external field is proposed. It is rigorously derived in [10] as \(\Gamma\)-limit of the full variational model for sufficiently large and thin samples within a certain parameter regime. Numerical simulations based on this model consist of a two step algorithm: First, the so-called effective magnetization \(m\) is computed under the relaxed constraint \(|m| \leq 1\). This represents the average magnetic charges due to external field and stray-field energies. Second, a stray-field free corrector that heuristically minimizes interface energy, i.e., wall energy, is added to obtain a physically relevant unit-length magnetization. This allows the reconstruction of certain mesoscopic patterns from the effective magnetization, i.e. explain the formation of the Landau ground states and corresponding distorted states that form under an adiabatically increased external field. One open question that is treated in the group is the rigorous analysis of the regularity of the effective magnetization.
In [11] we modified the model from [9] to allow for further applications. We replace the heuristic interface minimization in the second step of the algorithm by a more sophisticated selection criterion: As described in the Section on Wall configurations, an asymptotically correct energy density \(e(\alpha)\) of a wall (in dependence of the angle \(\alpha\)) is accessible. We thus first compute the energy density that corresponds to the desired parameter setting. Then, we solve a diffuse interface model (with numerical parameter \(\epsilon\ll 1\)) that asymptotically ensures:
- The corrector yields a physically relevant magnetization of unit length
- The interface energy of the walls is minimized
This approach is more flexible than the original selection criterion for the corrector function. It allows us to prescribe additional boundary data and thus effectively extend the range of applications. In [12] the authors describe the formation of a concertina pattern in rectangular films of moderate aspect ratio based on their experimental data. Apparently, hysteresis occurs due to the pinning of so-called wall-clusters at the boundary. This pinning can be explicitly included into our extended model; first numerical simulations show good qualitative agreement with experimental data. The extension of the model and the algorithms to capture the creation process of the whole concertina pattern is - among others - subject of ongoing research.
The simulation of the model from [9,11] requires sophisticated numerical schemes. In the dissertation [13], a barrier method is employed to compute the effective magnetization. In [14] a convergent penalty method is proposed and analyzed. The original selection criterion for the corrector requires to compute the viscosity solution to a modified Eikonal equation. This can be done by well-known level-set methods. The new selection criterion requires to solve a non-linear 4th order equation with very small parameter \(\epsilon\) weighting the leading order term. The development of suitable stable and reliable (adaptive) numerical schemes is a field of active research.
References
Antonio De Simone ; Robert V. Kohn ; Stefan Müller and Felix Otto: Recent analytical developments in micromagnetics
In: The science of hysteresis. Vol. 2 Physical modeling, micromagnetics, and magnetization dynamics / GiogioBertotti... (eds.)
Amsterdam : Elsevier [u. a.], 2006. - P. 269-381
MIS-Preprint: 80/2004
DOI: 10.1016/B978-012480874-4/50015-4
Felix Otto and Thomas Viehmann: Domain branching in uniaxial ferromagnets : asymptotic behavior of the energy
In: Calculus of variations and partial differential equations, 38 (2010) 1/2, p. 135-181
DOI: 10.1007/s00526-009-0281-y
Lukas Döring ; Radu Ignat and Felix Otto: A reduced model for domain walls in soft ferromagnetic films at the cross-over from symmetric to asymmetric wall types
In: Journal of the European Mathematical Society, 16 (2014) 7, p. 1377-1422
MIS-Preprint: 33/2012
DOI: 10.4171/JEMS/464
Lukas Döring ; RaduIgnat and FelixOtto: Crossover from symmetric to asymmetric transition layers
Rubén Cantero-Álvarez and Felix Otto: Critical fields in ferromagnetic thin films : identification of four regimes
In: Journal of nonlinear science, 16 (2006) 4, p. 351-383
DOI: 10.1007/s00332-004-0683-0
Rubén Cantero-Álvarez and Felix Otto: Oscillatory buckling mode in thin-film nucleation
In: Journal of nonlinear science, 16 (2006) 4, p. 385-413
DOI: 10.1007/s00332-004-0684-z
Rubén Cantero-Álvarez ; Felix Otto and Jutta Steiner: The concertina pattern : a bifurcation in ferromagnetic thin films
In: Journal of nonlinear science, 17 (2007) 3, p. 221-281
DOI: 10.1007/s00332-006-0805-y
Felix Otto and Jutta Steiner: The concertina pattern : from micromagnetics to domain theory
In: Calculus of variations and partial differential equations, 39 (2010) 1/2, p. 139-181
DOI: 10.1007/s00526-009-0305-7
Antonio DeSimone ; Robert V. Kohn ; Stefan Müller ; Felix Otto and Rudolf Schäfer: Two-dimensional modelling of soft ferromagnetic films
In: Proceedings of the Royal Society of London / A, 457 (2001) 2016, p. 2983-2991
MIS-Preprint: 30/2000
DOI: 10.1098/rspa.2001.0846
Antonio DeSimone ; Robert V. Kohn ; Stefan Müller and Felix Otto: A reduced theory for thin-film micromagnetics
In: Communications on pure and applied mathematics, 55 (2002) 11, p. 1408-1460
MIS-Preprint: 82/2001
DOI: 10.1002/cpa.3028
Lukas Döring ; Elias Esselborn ; Samuel Ferraz-Leite and Felix Otto: Domain configurations in soft ferromagnetic films under external field
In: Proceedings of MATHMOD 2012 - 7th Vienna International Conference on Mathematical Modelling, Vienna, February 15 - 17, 2012
Vienna: International Federation of Automatic Control, 2012. - P. 498-503
MIS-Preprint: 3/2012
DOI: 10.3182/20120215-3-AT-3016.00087
H. A. M. van den Berg and D. K. Vatvani: Wall clusters and domain-structure conversions
In: IEEE transactions on magnetics, 18 (1982) 3, p. 880-887
DOI: 10.1109/TMAG.1982.1061919
Jörg Drwenski: Numerical methods for a reduced model in thin-film micromagnetics
Dissertation, Universität Bonn, 2008
Samuel Ferraz-Leite ; Jens Markus Melenk and Dirk Praetorius: Numerical quadratic energy minimization bound to convex constraints in thin-film micromagnetics
In: Numerische Mathematik, 122 (2012) 1, p. 101-131
MIS-Preprint: 55/2011
DOI: 10.1007/s00211-012-0454-z
Presentations
- Pattern formation in micromagnetics (see PDF, 3.9 Mbyte)
- Overview and concertina pattern in thin films (see PDF, 5.2 Mbyte)
- Concertina pattern (see PDF, 2.0 Mbyte)
- Overview, concertina pattern, and branching (see PDF, 4.1 Mbyte)
- Stability of symmetric Neel walls in ferromagnetic thin films (see PDF, 105 Kbyte)
- Period of cross-tie wall in thin films, deformation of Landau state in thin films under external field (see PS, 8.4 Mbyte; see PS, 4.7 Mbyte; see PS, 13.0 Mbyte)
- Domain and wall patterns in ferromagnetic films (see MPG Yearbook 2011, German only)